The Prosik GmbH in cooperation with the Otto Junker GmbH has delivered an integrated process model for inductive heaters to Hydro Extrusion Poland located in Trzcianka. The model calculates the induced power to the billet and uses the resulting simulated temperature distribution inside the billet to control the process. The model improves the overall performance of the line by increasing the throughput as well as the temperature accuracy.
Introduction
Aluminium profiles are used in many applications such as door sills and pillars in the automotive sector or as construction elements. The base semi-finished products for extruding profiles are rods as shown in figure 1. The rods are cut into billets of lengths roughly between 500 mm and 1500 mm with diameters ranging from 200 mm to 360 mm depending on the particular process.
During the extruding process the billets are pressed through a die with a specific cross section. This process requires the billets to be heated to temperatures ranging from 400 °C up to 560 °C. If the temperature of the billet is too high the shape of the resulting cross section might be deformed. At lower temperatures the risk of defects increases and the required pressure to push the material through the die increases as well. Therefore, the precise heating of the billet is of great importance.
Inductive Billet Heating
Figure 2 shows one of the two inductive billet heaters in Trzcianka. One billet is currently inside the heater resting on the billet trough. After the heating is finished the billet trough moves out of the heater and the manipulator is used to take the billet to the press. As soon as the billet trough is cleared, the tilting trough is tilted and the fresh billet rolls onto the billet trough. A press-on thermocouple is uses to determine the billet temperature before the billet trough moves into the heater again. The tilting trough is loaded again with a fresh billet from the saw by the manipulator.
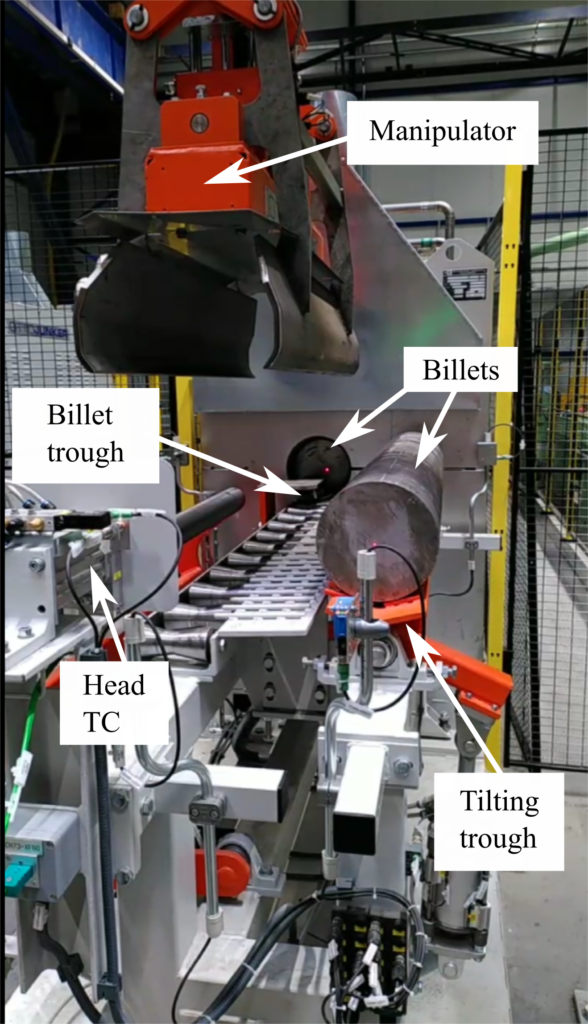
The heater in this case has four control sections. Each section contains an induction coil for heating and a pair of press-on thermocouples to measure the surface temperature of the billet. Based on this measurement the electrical power for heating in each section is controlled. This conventional approach has a few disadvantages.
Due to the fact that only the surface temperature of the billet is known it is not possible to determine the uniformity of the temperature distribution in radial direction. Therefore, the billet is held inside the heater longer than necessary to ensure that the core temperature also has reached the target value.
When measuring a surface temperature, the thermocouples should be aligned parallel to the surface in order to minimize errors in the measurement. For technical reasons the measurement in the heater has to be normal to the billet surface which is the worst case in regard to accuracy of the measurement.
Integrated Process Model
The solution to the described problems is the implementation of a process model that is integrated into the control system of the heater. Based on the electrical input of the induction coils the power induced to the billet surface is calculated for each section in real time. A FVM model of the billet takes the distribution of the induced power as an input to simulate the temperature distribution inside the billet. The model is directly connected to the PLC and generates target values for electrical power as output. This way, the process is controlled by the results of the model instead of the temperature measurements on the billet surface.
The validation of the model takes place on site as shown in figure 3. Billets are heated up and afterwards thermocouples are hammered in with parallel alignment to the surface, this minimizing the error in measurement. Based on this measurements the consideration of the efficiency factor of the induction coils and the losses during heating can be fine-tuned within the model.

Results
Using a process model has the great advantage of knowing the temperature distribution within the billet. This gives the opportunity to improve the control over the process. It allows the usage of an overshoot temperature. The billet surface is allowed to exceed the target billet temperature in order to speed up the heating process. With the model this overshoot temperature can be controlled precisely so that the average temperature of the billet equals the target temperature at the end of the process. During the transport of the billet to the press the remaining temperature gradient will decrease and the billet is at a uniform temperature.
Figure 4 shows the performance of the conventional control based on the thermocouple measurement (TC in red) compared to the performance of the model-based control (Model in blue). The conventional control heats up at maximum power until the surface temperature reaches the target value. After that the power is reduced to hold the surface temperature. If it exceeds the target value by 5 K the power is set to zero and surface cools down. If it falls below the target value the control starts heating again. This leads to the spikes in the power curve as shown below. Is the time between these spikes long enough, the billet is considered ready.
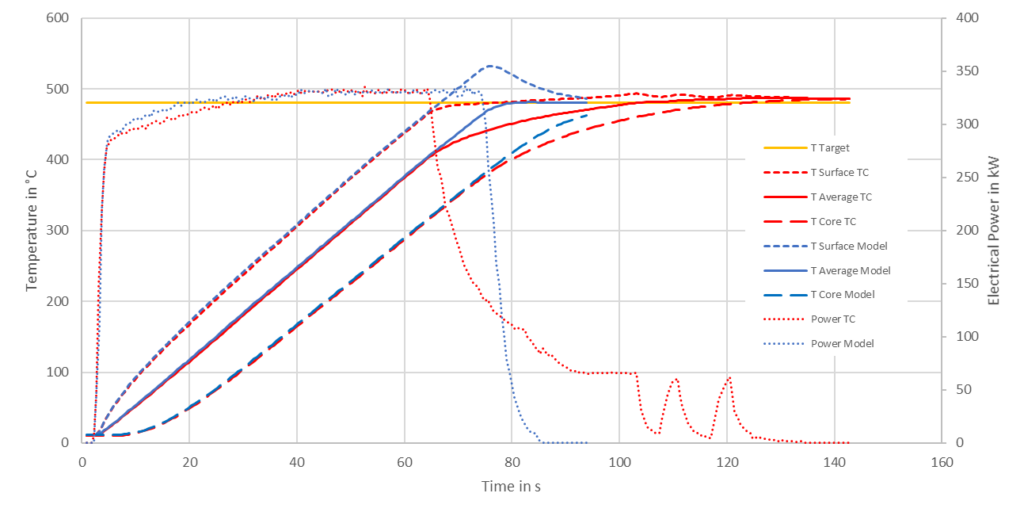
With the exact knowledge of the temperature distribution the model is able to keep the power at maximum level 10 to 15 seconds longer than the conventional control. The model takes the average temperature of the billet as control value and ensures that it reaches the target set point as quickly as possible. While doing that the power will only be reduced if the surface temperature would reach a critical level. After the average temperature reached the target value the billet is left in the heater for soaking only. If the temperature distribution is uniform enough the billet is considered ready for pressing.
As shown in figure 4 this approach significantly reduces the heating time. With the conventional control it takes about 140 seconds until the billet is ready for heating. The model approach needs only 95 seconds. Also, the temperature uniformity is better. This is because of the 2-point control used for the conventional approach. Depending on the point of time when the billet is called to the press it leads to a uncertainty of +-5 K in the billet temperature whereas the model always keeps the average temperature exactly at the target value.
Conclusion
Using an integrated process model to control the inductive heating process increases the throughput of the line as well as the temperature uniformity of the billets. It is possible to revamp existing lines from the conventional control to the model-based control. Besides better process performance the model also is able to generate easy to use data files that can be connected to data management systems in the context of data mining solutions.